The Designed “Just So” Universe
Originally published at Leadership UIntroduction
What does it mean on a human level for an engineer to design a product? On a grand scale, what would it mean to say that the universe is the product of an intelligent designer? And what evidence is there to support such a claim? What features of the universe suggest that a “home” has been carefully crafted for our benefit? William Paley in his classic Natural Theology (1802) provided evidence from both the physical sciences and the biological sciences for a designed universe, but the strength of his argument for design was limited by the scientific understanding of his time, and was subsequently called into question by Darwin’s theory of evolution. However, discoveries in astronomy and cosmology in the last half of the twentieth century have provided extremely compelling evidence for a designed universe. My purpose in this article is to provide a clear indication of what is meant by design and then summarize the factual basis from cosmology that our universe is indeed uniquely designed as a habitat for life in general and humans in particular.
What does an engineer do when he/she designs something?
To gain an understanding of what engineers do when they design products for consumers, let me first give a simple example of how we interact with our world when we prescribe physical events for a purpose. Suppose that I wanted to throw a water balloon from the Leaning Tower of Pisa in Italy to the plaza below, hitting a friend who is walking on the plaza (and hopefully, missing other tourists). Using the equations Newton discovered for motion and for gravitational attraction, I could describe the descent of the water balloon to the plaza below with the following simple algebraic relationship:
H(t) = h0 – (G m / r2 ) t2 /2 – v0 t (1)
where “G” is a universal constant which gives the strength of the gravitational force of attraction, “m” and “r” are the mass of the earth and the radius of the earth respectively, and “h0” and “v0” are the height in the tower from which I shall throw the balloon and the vertical velocity with which I throw the balloon. With these constants and initial conditions defined, I can then calculate the height of the water balloon; H(t) gives the calculated height of the water balloon as a function of time “t” from when I threw it. This equation may be used to guarantee that my balloon arrives at the plaza at just the right time to hit my friend. All I need to do is to determine at what time my strolling friend will be just below me in the plaza and then I can use Eq. 1 to determine the initial velocity with which I need to throw the balloon. Just dropping the balloon is also fine; I just set v0 = 0 and solve for the correct time to drop the balloon. The precision with which I must specify the velocity of the thrown balloon depends on the mathematical form of Eq. 1, the specified values for the universal constant G and the initial condition h0. With the simple mathematical form of Eq. 1 and the actual gravity force constant G and height of the Leaning Tower of Pisa, hitting my friend should be relatively easy.
The three factors which are essential in the prediction of the motion of the water balloon from the Leaning Tower to the plaza below illustrate the factors which are generally necessary to provide a purposeful outcomes in engineering work: (1) the mathematical form that nature takes (as illustrated by Eq. 1); (2) the values of the universal constants (G in Eq. 1); and (3) the boundary conditions, which include the height “h0” in the tower from which I throw the balloon and the initial velocity “v0” with which I throw the balloon. The terms “m” and “r” may be thought of as additional boundary conditions which are specific to the location of the tower on the surface of the earth (rather than some other location in the universe). The engineer has no control over the laws of nature and the mathematical forms that they take. Neither does the engineer have any control over the universal constants such as the gravity force constant. The engineer can only set the boundary conditions, which is done in engineering drawings, which specify exactly how a device will look when it has been fabricated.
Let us illustrate this design process with the requirements (or boundary conditions) which must be specified when an engineer designs an automobile. He/she must very carefully prescribe the conditions under which the chemical energy in gasoline is released and converted to torque on the wheels of the car. Each dimension for each engine part, for example, is critical so that the parts work together in a harmonious fashion. The absolute size and shape of the parts (as distinct from the relative size to fit with each other) depends on the forces to be developed and transmitted, which in turn depends on the weight of the car and the speed it should achieve in service. The weight depends on the size, which in turn depends on the number of passengers plus luggage the car will carry. These factors then determine the size of the cylinders and pistons to be used in the engine and the rate of gasoline injected into these cylinders. The brake and suspension systems independently have to be scaled to fit the weight requirements as does the specifications for the tires. Notice how many of the specifications are related to each other, and therefore, cannot be independently specified or assigned. The greater is this interdependence of specified boundary conditions, the more complex and demanding are the design requirements. Small errors in the specification of any of these requirements will produce either a car with very inferior performance, or worse yet, a car which does not function at all
In the next sections, we will explore whether the universe has these essential features which we associate with design. Purposeful outcomes in the natural world depend on (1) the mathematical form that nature assumes; (2) the values of the universal constants; and (3) the initial conditions, or boundary conditions. While engineers can only fix the boundary conditions, the suitability of the universe as a habitat for life in general and for human beings in particular depends on all three. Thus, we will consider how each of these requirements appears to be an essential in creating a suitable habitat for life.
The Remarkable Mathematical Form Nature Takes
Mathematics is an abstract intellectual activity that was begun in Greece in the 6th century B.C. by Pythagoras and developed by Euclid and Aristotle. Their studies began with straight lines and circles and extended to ellipses, created through sectioning cones. In the third century, B.C., Apollonias of Perga wrote eight monumental volumes devoted to these curves, describing their properties as “miraculous”. Yet it never occurred to these mathematicians that such beautiful abstract forms from mathematics were in fact descriptions of real world phenomena. Imagine the delight of Johannas Kepler (1571-1630) some eighteen centuries later when he discovered that the orbits of planets around the sun conformed to these same beautiful but abstract mathematical forms. Kepler noted,
“The chief aim of all investigations of the external world should be to discover the rational order and harmony which has been imposed on it by God and which He revealed to us in the language of mathematics.”
Galileo Galilei (1564-1642) observed that “the laws of nature are written by the hand of God in the language of mathematics”. Morris Kline in his book Mathematics: The Loss of Certainty (1980) notes that the religious mathematicians of the 16th and 17th century including Newton, Galileo, Kepler and Copernicus believed that the universe was orderly and thus described by mathematics because a rational God fashioned it that way. Kline says that these scientist/mathematicians believed that
“God had designed the universe, and it was to be expected that all phenomena of nature would follow one master plan. One mind designing a universe would almost surely have employed one set of basic principles to govern all related phenomena.”
Even these devoted Christian scientists would no doubt be surprised to learn that all of the incredibly diverse phenomena we see in nature are the result of such a small number of physics laws, each of which assumes such a simple mathematical form, that they can all be written on one side of one sheet of paper, as seen in Table 1.
Table 1: Fundamental Laws of Nature
Mechanics (Hamilton’s Equations)
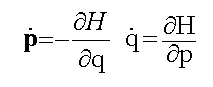
Electrodynamics (Maxwell’s Equations)
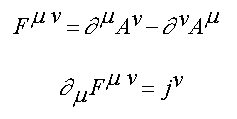
Statistical Mechanics (Boltzmann’s Equations)
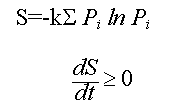
Quantum Mechanics (Schrödinger’s Equations)

General Relativity (Einstein’s Equation)
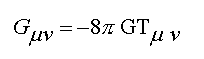
Physicist Eugene Wigner in a widely quoted paper entitled “The Unreasonable Effectiveness of Mathematics in the Physical Sciences” (Comm. Pure and Appl. Math, 1960) notes that the scientist often takes for granted the remarkable, even miraculous effectiveness of mathematics in describing the real world. To quote Wigner,
“The enormous usefulness of mathematics is something bordering on the mysterious……There is no rational explanation for it…….The miracle of the appropriateness of the language of mathematics for the formulation of the laws of physics is a wonderful gift which we neither understand nor deserve……”
Albert Einstein in a letter to a friend (1956, Lettres a Maurice Solovine) commenting on the mathematical comprehensibility of the world noted,
“You may find it strange that I consider the comprehensibility of the world to the degree that we may speak of such comprehensibility as a miracle or an eternal mystery. Well, a priori one should expect a chaotic world, which cannot be in any way grasped through thought… The kind of order created, for example, by Newton’s theory of gravity is of quite a different kind. Even if the axioms of the theory are posited by a human being, the success of such an enterprise presupposes an order in the objective world of a high degree, which one has no a priori right to expect. That is the miracle which grows increasingly persuasive with the increasing development of knowledge.”
Unlike Einstein and Wigner but in keeping with Newton and his contemporaries, many modern physicists consider the remarkable mathematical form which nature assumes to be evidence for the existence of an intelligent designer/creator, or God rather than a mystery. For example, the distinguished Russian physicist Alexander Polykov notes that, “We know that nature is described by the best of all possible mathematics because God created it.” Australian astrophysicist Paul Davies says, “The equations of physics have in them incredible simplicity, elegance and beauty. That in itself is sufficient to prove to me that there must be a God who is responsible for these laws and responsible for the universe.”
Finally, mathematical form alone is insufficient to guarantee a universe that is a suitable habitat. The particular mathematical form is also critical. For example, it is essential that the mathematical form provide for stable systems at the atomic and cosmic level. The solutions to Hamilton’s equations for non-relativistic, Newtonian mechanics and for Einstein’s theory of general relativity in Table 1 for a sun with planets is unstable unless the gravitational potential energy is proportional to r-1, a requirement which is only met for a universe with three spatial dimensions. For the solution to Schrodinger’s equation (Table 1) for the hydrogen atom to give stable, bound energy levels, again a universe with three (or fewer) spatial dimensions is required. Maxwell’s equations (Table 1) are also only valid for a three-(spatial) dimensional universe. Furthermore, Courant and Hilbert (1962, Methods of Mathematical Physics) have found that high-fidelity transmission of electromagnetic or acoustic signals is optimized in our three-dimensional universe, saying,
“…our actual physical world, in which acoustic or electromagnetic signals are the basis of communication seems to be singled out among the mathematically conceivable models by simplicity and harmony.”
In summary, it is clear that the specific mathematical character of our universe is essential for it to be a suitable habitat for life; yet the reason that nature has this precise mathematical form is problematic from a naturalistic metaphysics.
The Mystery of the Cosmological Constants
There are certain universal constants that are an essential part of our mathematical description of the universe. A partial list is found in Table 2 and includes Planck’s constant “h”, the speed of light “c”, the gravity force constant “G”, the mass of the proton, electron, and neutron, the unit charge for the electron or proton, and the weak force, strong nuclear force and electromagnetic coupling constants, and Boltzmann’s constant, k. When cosmological models were first developed in the mid-twentieth century, it was naively assumed that the selection of a given set of constants was not critical to the formation of a suitable habitat for life. Subsequent parametric studies which systematically varied the constants have shown that changes in any of the constants produces a dramatically different universe which is unsuitable for life of any imaginable type.
A large number of books have been written in the past ten years to summarize this surprising feature of our universe; namely, that the universal constants have to be “just so” to have a universe suitable for life. A partial list includes The Anthropic Cosmological Principle by Barrow and Tipler (1986), Universes by John Leslie (1989), The Accidental Universe (1982), Superforce (1984), and The Cosmic Blueprint (1988) by Davies, Cosmic Coincidences by Gribbin and Rees, The Anthropic Principle by Reinhard Breuer (1991), Universal Constants in Physics by Gilles Cohen-Tannoudji (1993), The Creation Hypothesis edited by J.P. Moreland (1994) and Mere Creation edited by William Dembski (1998). I will illustrate this “just so” requirement for the various universal constants and properties of matter by sharing several examples.
Table 2: Universal Constants
Universal Constants
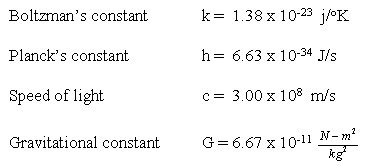
Mass of Elementary Particles
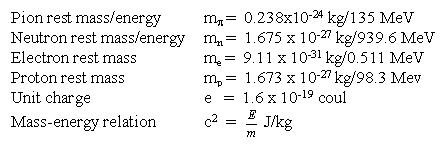
Fine Structure of Constants

Physical Fine Structure Constants — The four forces in nature may each be expressed in a dimensionless fashion to allow their relative strengths as they act in nature to be expressed in a way that facilitates comparison. These are summarized in Table 2, and are seen to vary by 1041, or 41 orders of magnitude (10 with 40 additional zeros after it). Yet modest changes in any of these constants produce dramatic changes in the universe which render it unsuitable for life. Several examples will serve to illustrate this “fine tuned” nature of our universe.
The relative magnitude of the gravity force and the electromagnetic force has been found to be crucial for multiple reasons. Note from Table 2 that the electromagnetic force is 1038 times stronger than the gravity force. It is the force of gravity that draws protons together in stars causing them to fuse together with a concurrent release of energy. The electromagnetic force causes them to repel. Because the gravity force is so weak by comparison to the electromagnetic force, the rate at which stars “burn” by fusion is very slow, allowing the stars to provide a stable source of energy over a very long period of time. If this ratio of strengths had been 1032 instead of 1038 (i.e., gravity were much stronger), stars would be a billion times less massive and would burn a million times faster.
The frequency distribution of electromagnetic radiation produced by the sun is also critical, as it needs to be tuned to the energies of chemical bonds on earth. If the photons of radiation are too energetic (too much ultraviolet radiation), then chemical bonds are destroyed and molecules are unstable; if the photons are too weak (too much infrared radiation), then chemical reactions will be too sluggish. The radiation produced is dependent on a careful balancing of the electromagnetic force (alpha-E) and the gravity force (alpha-G), with the mathematical relationship including (alpha-E)12, making the specification for the electromagnetic force particularly critical. On the other hand, the chemical bonding energy comes from quantum mechanical calculations that include the electromagnetic force, the mass of the electron, and Planck’s constant. Thus, all of these constants have to be sized relative to each other to give a universe in which radiation is tuned to the necessary chemical reactions that are essential for life.
Another interesting fine tuning coincidence is that the emission spectrum for the sun not only peaks at an energy level which is idea to facilitate chemical reaction but it also peaks in the optical window for water. Water is 107 more opaque to ultraviolet and infrared radiation than it is to radiation in the visible spectra (or what we call light). Since living tissue in general and eyes in particular are composed mainly of water, communication by sight would be impossible were it not for this unique window of light transmission by water being ideally matched to the radiation from the sun. Yet this matching requires carefully prescribing the values of the gravity and electromagnetic force constants as well as the Planck’s constant and the mass of the electron.
Next consider the strength of the nuclear strong force. The most critical element in nature for the development of life is carbon. Yet, it has recently become apparent that the abundance of carbon in nature is the result of a very precise balancing of the strong force and the electromagnetic force, which determine the quantum energy levels for nuclei. Only certain energy levels are permitted for nuclei and these may be thought of as steps on a ladder. If the mass-energy for two colliding particles results in a combined mass-energy which is equal to or slightly less than a permissible energy level on the quantum “energy ladder”, then the two nuclei will readily stick together or fuse on collision, with the energy difference needed to reach the step being supplied by the kinetic energy of the colliding particles. If this mass-energy level for the combined particles is exactly right, or “just so”, then the collisions are said to have resonance, which is to say a high efficiency of collisions giving fusion of the colliding particles. If the combined mass-energy results in a value which is slightly higher than one of the permissible energy levels on “energy ladder”, then the particles will simply bounce off of each other rather than sticking together, or fusing. Hoyle (1970) predicted that the existence of the unknown resonance energy level for carbon, and it was subsequently found to exist. The fusion of helium and beryllium give a mass-energy value that is 4% less than the resonance energy in carbon, which is easily made up by kinetic energy. Equally important was the discovery that the mass-energy for the fusion of carbon with helium was 1% greater than quantum energy level on the “energy ladder” for oxygen, making this reaction quite unfavorable. Thus, almost all beryllium is converted to carbon, but only a small fraction of the carbon is immediately converted to oxygen. These two results require the specification of the relative strength of the strong force and the electromagnetic force to within ~1%, which is truly remarkable in view of their large absolute values and difference of a factor of 100X, as seen in Table 2.
In a more general vain, a 2% increase in the strong force relative to the electromagnetic force leaves the universe with no hydrogen, no long-lived stars which burn hydrogen, and no water (which is a molecule composed of two hydrogen atoms and one oxygen atom), the ultimate solvent for life. A decrease of only 5% in the strong force relative to the electromagnetic force would prevent the formation of deuterons from combination of protons and neutrons, which would in turn prevent the formation of all the heavier nuclei through fusion of deuterons to form helium, helium fusion with helium to form beryllium and so forth. Rozental (1980) estimates that the strong force had to be within 0.8 and 1.2 times its actual strength for there to be deuterons and all elements of atomic weight 4 or more.
If the weak force coupling constant (see Table 2) were slightly larger, neutrons would decay more rapidly, reducing the production of deuterons, and thus, of helium and elements with heavier nuclei. On the other hand, if the weak force coupling constant were slightly weaker, the big-bang would burn almost all of the hydrogen into helium with the ultimate outcome being a universe with little or no hydrogen and many heavier elements instead. This would leave no long-term stars and no hydrogen containing compounds, especially water. Breuer (1991) notes that appropriate mix of hydrogen and helium to provide hydrogen containing compounds, long term stars, and heavier elements is approximately 75% hydrogen and 25% helium, which is just what we find in our universe. This balance requires that the weak force coupling constant (alpha-W) be proportioned to the gravity force coupling constant (alpha-G) in the following proportion: (alpha-W)4 ~ (alpha-G), which one can see from Table 2 is in fact satisfied.
This is only an illustrative but not exhaustive list of examples of cosmic coincidences that clearly demonstrate that the four forces in nature have been very carefully scaled to give a universe that provides long-term sources of energy and a variety of atomic building blocks which are necessary for life. Many other examples are summarized in the books cited, some of which are quite amusing. For example, a much larger value of gravity would give a greater likelihood that when we fall down, we would break due to the much greater gravity force. But what should we think about the elementary particles and other universal constants such as the speed of light and the Planck’s constant? Do they also have to be very precisely specified?
Masses of Elementary Particles and Other Universal Constants — It has been surprising to learn that the masses of the elementary particles must also be very carefully specified relative to each other and also to the forces in nature. For example, Stephen Hawking (1980) has noted that the difference in the mass of the neutron and the mass of the proton must be approximately equal to twice the mass of the electron. The mass- energy of the proton is 938.28MeV, the mass-energy of the electron is 0.51MeV and the neutron weighs in at 939.57 MeV. If the mass-energy of the proton plus the mass-energy of the electron were not slight smaller than the mass-energy of the neutron, then electrons would combine with protons to form neutrons, with all atomic structure collapsing, leaving a world of neutron only. If this difference were much larger, then neutrons would all decay into protons and electrons, leaving a world of hydrogen only, since neutrons are necessary to allow protons to combine to build up heavier nuclei and the associated elements. As things are, the neutron is just heavy enough to ensure that the big-bang yields one neutron to every seven protons, allowing for an abundant supply of hydrogen for star fuel and enough neutrons to build up the heavier elements in the universe. Again, the precise relative values for the masses of these elementary particles are seen to be critical to provide a universe with long-term sources of energy and elemental diversity.
Examples of other essential relationships for the masses of elementary particles to permit the formation of heavier elements in nature have been provided by Brandon Carter (1970) as follows: the strong force must be related to the mass of the neutron and the mass of the pion by (alpha-S)2 ~ 2 (neutron mass/pion mass); the electromagnetic fine structure constant (alpha-E) ~ [(neutron mass) – (proton mass)] / (pion mass); and the strong force fine structure must obey (alpha-S)2 ~ 1 / (9 alpha-E). Table 2 may be used to show that each of these requirements is indeed satisfied. It is remarkable that these relationships are all satisfied, despite the fact that these masses and forces appear to be independent in their assignment and not causally connected. Additional requirements could also be stated for h, k, c, and other constants.
We will conclude this section of cosmological coincidences by allowing several distinguished scientists give their significant to the observations summarized above. For example, Freeman J. Dyson says,
“As we look out into the universe and identify the many accidents of physics and astronomy that have worked to our benefit, it almost seems as if the universe must in some sense have known that we were coming.”
Nobel laureate Arno Penzias makes this observation about the enigmatic character of the universe,
“Astronomy leads us to an unique event, a universe which was created out of nothing and delicately balanced to provide exactly the conditions required to support life. In the absence of an absurdly-improbable accident, the observations of modern science seem to suggest an underlying, one might say, supernatural plan.”
Sir Fred Hoyle, famous British astronomer who early on (1951) argued that the coincidences were just that, coincidences, by 1984 had changed his mind, as is evident from this quotation:
“Such properties seem to run through the fabric of the natural world like a thread of happy coincidences. But there are so many odd coincidences essential to life that some explanation seems required to account for them.”
The Remarkable Requirements for Initial Conditions
The specific mathematical form that nature takes and the highly specific values of the various universal constants and masses of elementary particles alone cannot account for our habitat and for life. All of this could have been done in the elegant way that it has been done, as described above, and life would still not have occurred if the boundary conditions at certain critical points had not been properly set. In this section, the initial conditions for the big bang will be discussed. A similar problem for the origin of life and possibly for the Cambrian explosion also exist, but the discussion of these will be left to more detailed articles elsewhere in this special edition.
The fundamental boundary value (or initial condition) problem with the big bang is the criticality of the initial velocity. If this velocity is to fast, the matter in the universe expands too quickly and never coalesces into planets, stars, and galaxies. If the initial velocity is too slow, the universe expands only for a short time and then quickly collapses under the influence of gravity. Well-accepted cosmological models tell us that the initial velocity must be specified to a precision of 1 / 1055. This requirement seems to overwhelm chance and has been the impetus for creative alternatives, most recently the new inflationary model of the big bang. However, inflation itself seems to require fine-tuning for it to occur at all and for it to yield irregularities neither to small nor to large for galaxies to form. Early on it was estimated that two components of an expansion-driving cosmological constant must cancel each other with an accuracy better than 1 part in 1050. More recently in Scientific American (January 1999), the required accuracy is stated to be 1 part in 10123. Furthermore, the ratio of the gravitational energy to the kinetic energy must equal to 1.00000 with a variation of 1 part in 100,000. This is an active area of research at the moment and these values may change over time. However, it appears that the essential requirements of very highly specified boundary conditions will be present in whatever model is finally confirmed for the big bang origin of the universe.
Summary
My initial example of design was a very simple one involving one physical law, one universal constant, and two initial conditions which could be prescribed in such a way that my water balloon would arrive on the plaza of the Leaning Tower of Pisa just in time to hit my strolling friend. This is a relatively easy design problem. However, for the universe to have stars which generate elemental diversity, provide long-term sources of energy of a suitable wavelength of radiation to facilitate chemical reactions, and satisfy many other requirements for a suitable habitat for life and for the origin of life, the mathematical form of the laws of nature, the 19 universal constants (not all of which are listed in Table 2), and many initial conditions have to be “JUST SO“. Many of these requirements are interrelated. For example, the initial velocity requirement is related to the strength of the gravity force. There are so many different requirements that are interrelated, it seem difficult to imagine how all of these “accidentally” happened to be exactly what they need to be. Because of the many cross constraints, it appears unlikely that there is an alternative set of values for these constants which would “work”. Furthermore, the necessary values range over thirty orders of magnitude (1030), making their accidentally correct “selection” all the more remarkable. It is quite easy to understand why so many scientists have changed their minds in the past 30 years, agreeing that it takes a great deal of faith to believe the universe can be explained as nothing more than a fortuitous cosmic accident. Evidence for an intelligent designer becomes more compelling the more we understand about our carefully crafted habitat.